Project Arnold Reusken

Summary
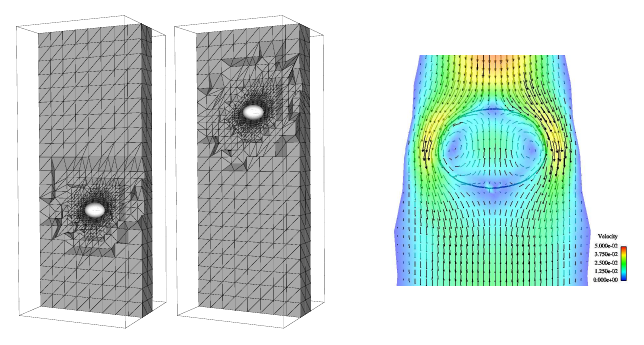
In this project we develop, analyze and implement numerical methods for the simulation of two-phase incompressible flows with mass transport. The mass transport of a dissolved species is modeled by a time-dependent convectiondiffusion equation combined with appropriate coupling conditions at the interface. The solution of this equation is in general discontinuous across the unkown interface between the two phases and in our applications this problem is often convection-dominated. This causes severe numerical difficulties. We plan to develop new stable and efficient finite element discretization methods for this transport equation. Both a rigorous error analysis and the application of these methods will be investigated. The methods will be implemented in a software package (DROPS) that is available in our group. The numerical simulation of fluid dynamics and mass transport with the DROPS package will be validated in collaboration with a partner from an experimental project on Taylor flows in mini-channels.